How to define the tangent function using the unit circle how to transform the graph of tangent functions examples and step by step solutions a series of free high school trigonometry video lessons how to graph the tangent function on the coordinate plane using the unit circle how to determine the domain and range of the tangent function reciprocal identity. Lets start with the basic sine function f t sin t.
If you change your a then youll also be changing your amplitude.
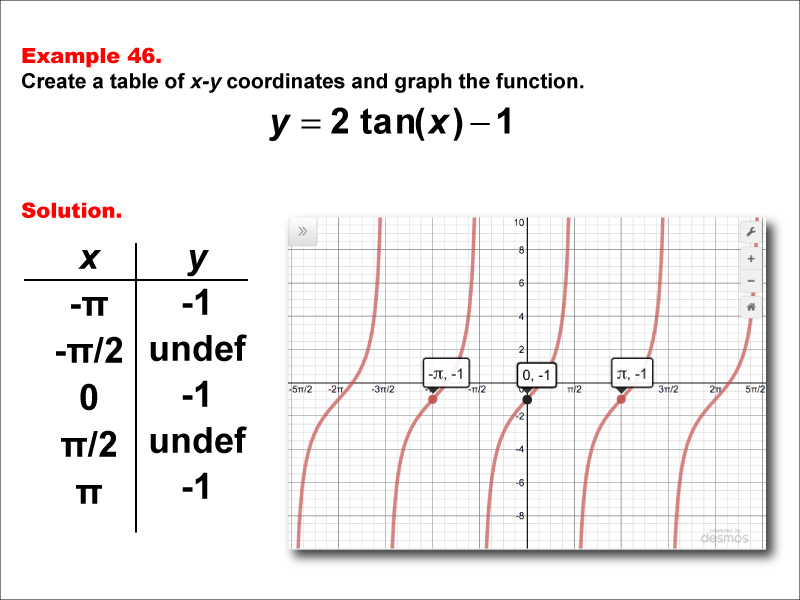
How to graph tangent functions.
The graph domain range and vertical asymptotes of these functions and other properties are examined.
Then draw in the curve.
The asymptotes for the graph of the tangent function are vertical lines that occur regularly each of them p or 180 degrees apart.
Let us make a table over one period p2 p2 using the variable t.
For graphing draw in the zeroes at x 0 p 2p etc and dash in the vertical asymptotes midway between each zero.
Page 1 of 2 141 graphing sine cosine and tangent functions 831 graphing sine cosine and tangent functions graphing sine and cosine functions in this lesson you will learn to graph functions of the form yasinbxand yacosbxwhere aand bare positive constants and xis in radian measure.
Recall that the tangent function can be defined as.
The amplitude really only applies to the sine and cosine functions.
Find the vertical asymptotes so you can find the domain.
How to graph a tangent function.
The equations of the tangents asymptotes are all of the form where n is an integer.
Without an a value the amplitude for your sine and cosine functions is 1.
As you can see the tangent has a period of p with each period separated by a vertical asymptote.
In order to.
The concept of amplitude doesnt really apply.
The graphs of all sine and cosine functions are related to the graphs of.
Here we will use radians.
You can see this on the graphs of these functions since the graph goes up to 1 at its highest point.
They separate each piece of the tangent curve or each complete cycle from the next.
Graphing tangent function the trigonometric ratios can also be considered as functions of a variable which is the measure of an angle.
Determine values for the range.
Solution to example 2.
A step by step tutorial on graphing and sketching tangent functions.
The closer you get to the values.
These steps use x instead of theta because the graph is on the x y plane.
Let t 2 x p4.
This angle measure can either be given in degrees or radians.
This function has an amplitude of 1 because the graph goes one unit up and one unit down from the midline of the graph.
This function has a period of 2p because the sine wave repeats every 2p units.
No comments:
Post a Comment